27 Oct Hydrodynamics of Hypersonic Jets
The hydrodynamics of astrophysical jets: scaled experiments and numerical simulations
Abstract
Astrophysical jets are collimated outflows of matter and fields propagating at supersonic, and often relativistic, velocities. Jets are ubiquitous in the Universe, in fact they are observed emerging from protostellar objects, from stellar X-ray binaries, as well as from supermassive black holes located at the center of active galaxies. Jets are thougth to originate from a central object surrounded by a magnetized accretion disk, there a significant fraction of the inflowing matter is ejected along the rotation axis by magnetohydrodynamic processes. The studies about launching, propagation and stability of jets are carried out by analytical and semi-analytical means, by numerical simulations and laboratory experiments. The physics that encompasses all classes of astrophysical jets is magnetohydrodynamics. We have conceived laboratory and three-dimensional numerical mid-long term simulations of hypersonic Newtonian jets, in the hydrodynamic limit, which meet the two main scaling parameters for protostellar jets, i.e. the formation Mach number and the ambient/jet density ratio. In the laboratory experiments jets are generated, in a quasi-isentropic way, with the property of being either lighter or heavier than the ambient. These simulations show a few of the universal morphological properties observed in the intermediate-far field of protostellar jets. Both experimental and numerical simulations highlight that a high collimation is achieved and maintained for the two kind of jets, at least within the experimental limits. Moreover, light jets show aligned bright high density regions in form of knots with quasi-regular spacing.
It is noticeable that even the intense interaction with the exteriors experienced by a light jet is not sufficient to spoil its collimation – which thus is an expression of the prevailing inertial effects. These results show that high Mach number jets survive and maintain the collimation for several jet radii even without the confining effects of magnetic fields, as suggested for astrophysical jets in many instances. The good qualitative agreement between laboratory and numerical results is, in a more general context, a significant validation for the numerical schemes adopted for the treatment of highly compressible flows in presence of strong shocks. See Abstract.In this study we focused on the propagation of hypersonic hydrodynamic jets (Mach number >5) in a laboratory vessel and make comparisons with numerical simulations of axially symmetric flows with the same initial and boundary conditions. The astrophysical context is that of the jets originating around young stellar objects (YSOs).
Aims: In order to gain a deeper insight into the phenomenology of YSO jets, we performed a set of experiments and numerical simulations of hypersonic jets in the range of Mach numbers from 10 to 20 and for jet-to-ambient density ratios from 0.85 to 5.4, using different gas species and observing jet lengths of the order of 150 initial radii or more. Exploiting the scalability of the hydrodynamic equations, we intend to reproduce the YSO jet behavior with respect to jet velocity and elapsed times. In addition, we can make comparisons between the simulated, the experimental, and the observed morphologies.
Methods: In the experiments the gas pressure and temperature are increased by a fast, quasi-isentropic compression by means of a piston system operating on a time scale of tens of milliseconds, while the gas density is visualized and measured by means of an electron beam system. We used the PLUTO software for the numerical solution of mixed hyperbolic/parabolic conservation laws targeting high Mach number flows in astrophysical fluid dynamics. We considered axisymmetric initial conditions and carried out numerical simulations in cylindrical geometry. The code has a modular flexible structure whereby different numerical algorithms can be separately combined to solve systems of conservation laws using the finite volume or finite difference approach based on Godunov type schemes.
Results: The agreement between experiments and numerical simulations is fairly good in most of the comparisons. The resulting scaled flow velocities and elapsed times are close to the ones shown by observations. The morphologies of the density distributions agree with the observed ones as well.
Conclusions: The laboratory and the simulated hypersonic jets are all pressure matched, i.e. their axial regions are almost isentropic at the nozzle exit. They maintain their collimation for long distances in terms of the initial jet radii, without including magnetic confinement effects. This yields a qualitatively good agreement with the observed YSO jet morphologies. It remains to be seen what happens when non-axially symmetric perturbations of the flow are imposed at the nozzle, both in the experiment and in the simulation.
For more detail please see the The hydrodynamics of astrophysical jets: scaled experiments and numerical simulations
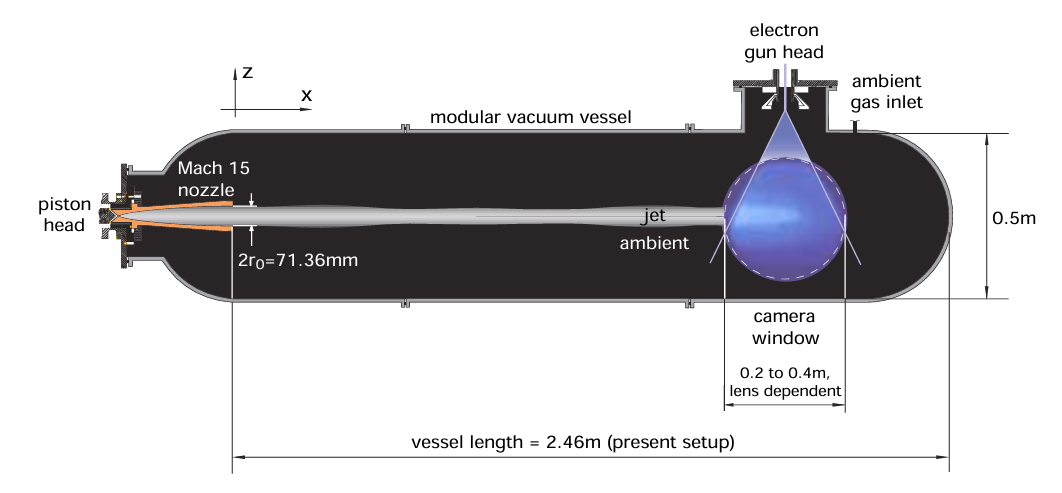
The experimental setup
The laboratory experiment was carried out in an apparatus designed for the study of hypersonic jets (Belan et al 2004, 2008, 2010). The flows under test are jets obtained from a de Laval nozzle that propagate along the longitudinal axis of a cylindrical vacuum vessel. In this apparatus, it is possible to use different gases for the jet and the surrounding ambient in the vessel, which allow a wide variation of the nominal density ratio η as an independent parameter. The gases involved in these experiments are helium, argon, xenon and air. The vessel is modular: five different sections are available for mounting and the total length can vary from 1.4 to 4.1 m, with a constant diameter of 0.5 m. The vessel was mounted in a three-section configuration for this work. Several nozzles designed for Mach numbers ranging from 5 to 20 are available. In this study, the jets were produced using a nozzle with a nominal Mach number of 15. The gas flow in the nozzle is driven by a fast piston system, which is capable of producing the right gas stagnation conditions. The diameter of the nozzle exit section is 2r0 = 71.36 mm; this is also the nominal diameter of the jets. The vessel diameter is 14 times larger than the diameter of the jets. An electron gun, equipped with a deflection system, creates an electron sheet. This sheet intercepts the jet and generates a plane fluorescent section of the flow, which is then acquired by a fast CMOS camera equipped with an image intensifier. The electron gun and camera can be mounted onto several ports and optical windows. Here they have been mounted in such a way as to acquire the field between nearly 24 and 28 jet diameters from the nozzle exit. The present configuration of the apparatus is sketched in Figure 1. For details of the flow visualization technique, see the Astrophysical jets: insights into long-term hydrodynamics.
Fig. 1. Experimental setup. The total vessel length depends on the number of assembled sections. The set of de Laval nozzles used in this work is shown on the left. All the nozzles have the same converging section and throat (radius = 1 mm), whilst the diverging section depends on the Mach number of the design. The output radii rn are 12.0, 35.7, and 60.9 mm.
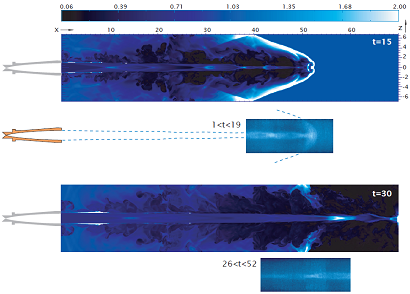
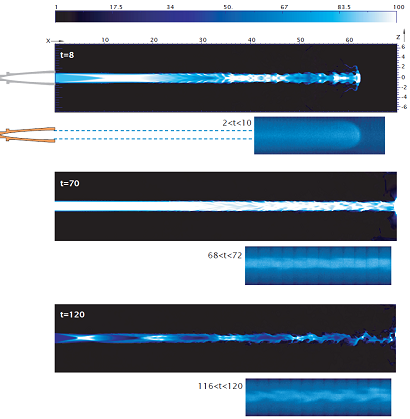
This section presents the different morphologies observed at some significant density ratios and Mach numbers, selected as Figure 2-4 which show three sets of jets, chosen in order to present slightly underdense, slightly overdense, and overdense jets. For each set, three different Mach numbers are selected, and for each jet the laboratory visualization is compared with the numerical simulation. In the visualizations, thanks to the electron beam technique, the light intensity is proportional to the local density, whereas in the corresponding numerical simulations the density maps are intentionally produced for the sake of comparison with the experiment, even if the simulations may easily produce maps for many other quantities.
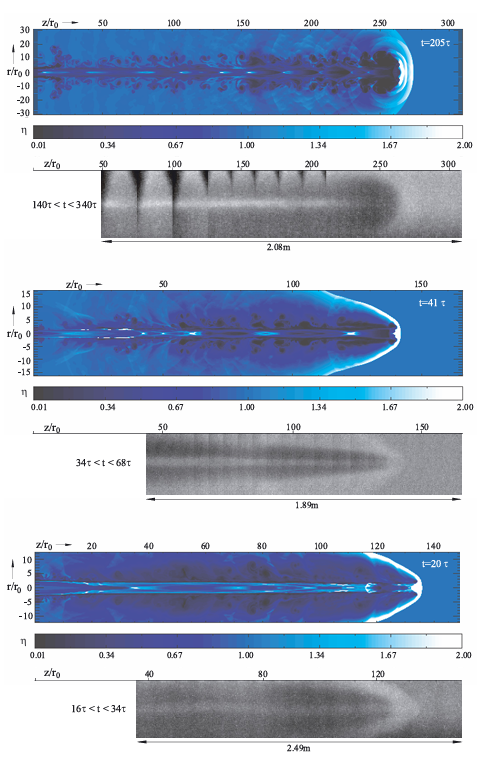
Figure 2. Set of three slightly underdense jets of He in Xe: from top to bottom, η = 0.85, 0.9, and 0.9, Mach number = 10.0, 13.5, and 18.0. Length is expressed in r0 units (effective or reference jet radius) and time in τ units, i.e sound crossing time over jet radius. The numerical density scale refers to the unperturbed ambient density.
A simple quantitative comparison between experiments and simulations can be made by measuring the jet head velocity Vexp. The results are in Table 1 and agree well with the numerical results Vnum, slightly less well at the lowest Mach number. For the experimental images, the results were obtained by means of the above mentioned correlation technique.
Table 1. Head velocities for Fig. 2.
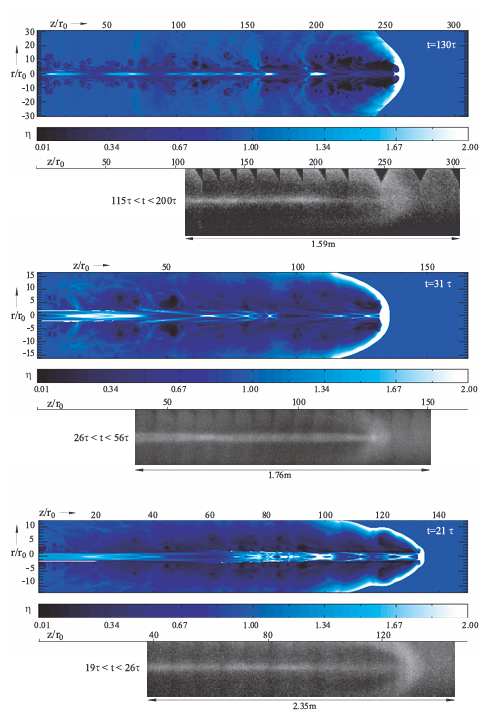
Figure 3. Set of three slightly overdense jets of He in Xe: from top to bottom, η=1.2, 1.35, and 1.4, Mach number=11.0,16.0, and 18.0.
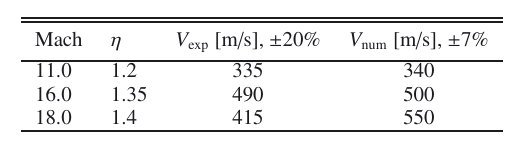 Table 2. Head velocities for Fig.3
Table 2. Head velocities for Fig.3

Figure 4. Set of three overdense jets of He: from top to bottom, ambient
is air, and He; η=4.4, 4.6, and 5.4; Mach number=7.0, 14.3,
and 17.9.

Table 3. Head velocities for Fig.4.
Conclusions
The experimental facility and instrumentation have been shown to fit the experimental requirements and to give valid results for the aim they were designed and assembled for. Both experiments and numerical simulations have shown detailed aspects of the head bow shock, its bluntness versus Mach number and the shape of the cocoon as a function of the density ratio. In this sense the use of gases of strong density difference, such as helium and xenon has been very important. We note that both the experiments and then numerical simulations agree well in most cases. Moreover, the Euler similar scaled flows that were produced in the laboratory are characterized by velocities that also agree well with YSO jets values. The physical characteristics of simulated jets, i.e. head and jet velocities, bow-shock, and cocoon morphologies that we discuss in the paper are intentionally limited to the ones that can be obtained either by direct measurements or that are derived in the laboratory experiments, and are thus subjects of comparison. These experiments suggest once again that the PLUTO code may be considered a valid tool for HD simulations of astrophysical jets, as a useful tool for fur the investigations. Physical elements, such as radiative cooling which is important in determining the jet emission details, and magnetic fields which are important in determining the out flow launching, are absent in our investigation. However, we can say that the basic features of the jet dynamics are well described by the HD treatment alone, and it is therefore reasonable to assume that fluid dynamics may explain the major characteristics of the behaviour of YSO gas jets with respect to morphology and collimation. We are almost at the limits of the experimental instrumentation capabilities. It is difficult to imagine cameras with higher performance in resolution or sensitivity and acquisition time, or to imagine increasing the electron beam characteristics beyond 2m without increasing the beam width, or voltage, going into X-ray emission. A significant change could be achieved by using different measurements techniques, like molecular tagging velocimetry or laser interferometry; of course these techniques might be considered radical, long term modifications of the existing set up. Another progress in the facility would be to increase the temperature of the gas jet. The facility now has the advantage of being economically manageable and low-energy, while the major modifications to the whole system needed to heat the gas jet would require a very high cost.
Longterm dynamics of rarefied jets
– Visualization of density and concentration distributions inside and outside the barrel shock in underexpanded hypersonic jets. We show that the jet growth clearly depends on the sign of the density gradient between the gas in the jet and that in the ambient surroundings.
For more details please see (Physical Review E 2010, Experiments in Fluids 2008, Astrophysics and Space Science 293, 2004)
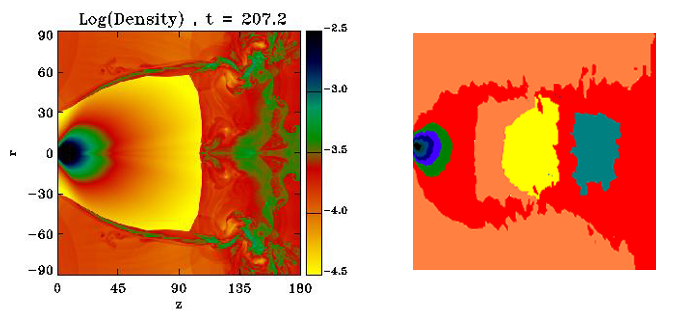
Figure 1. A new fluorescent image analysis ù procedure to determine the distribution of species concentration and density in a gas flow is proposed (Exp. Fluids, 2008). The fluorescent emission is due to the excitation of atoms/molecules of a gas that is intercepted by an electron blade.
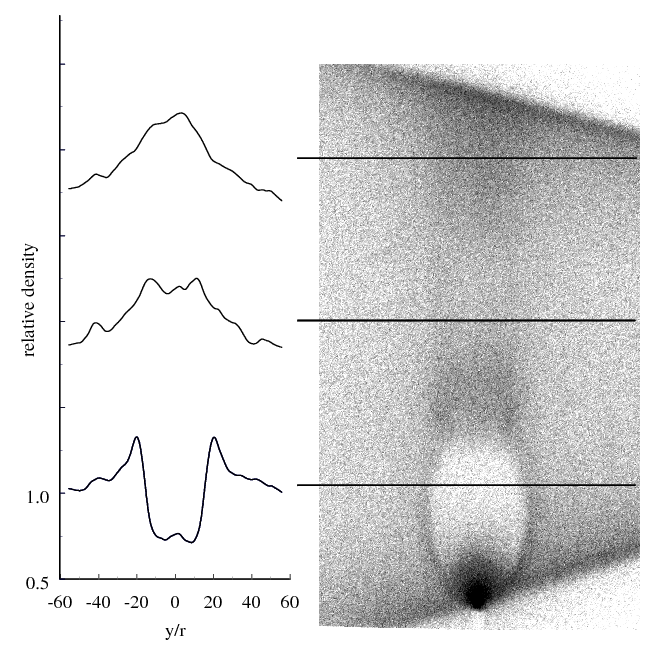
Figure 2. Extraction of the density profiles from the recorded images by means of a new fluorescent image analysis procedure (Exp. Fluids, 2008). Left: air jet. Right: He/Ar jet.
Figure 3. Comparison between numerical simulations and laboratory experiments