04 Feb Turbulent Mixing
Small-Scale Anisotropy in Turbulent Shear-less Mixing
The Philofluid Research Group is dedicated to advancing the understanding of turbulence dynamics through both laboratory and numerical investigations. Our focus lies in the small-scale features of turbulence, which involve a complex range of scales, each governed by distinct physics. While energy dissipation occurs at smaller scales, it remains interconnected with the large-scale dynamics of the system, indicating the possibility of long-range interactions.
One key phenomenon observed in turbulent flows is small-scale anisotropy, often characterized by velocity derivative statistics. In particular, we have studied the velocity derivatives in shearless turbulent mixing during its temporal decay, a mild form of inhomogeneity where near-isotropic conditions can be expected. The shearless turbulent mixing layer, a simple example of an inhomogeneous turbulent flow, emerges from the interaction of two isotropic turbulence regions without a mean shear flow, resulting in no turbulent kinetic energy production or mean convection.
Our research highlights how statistical turbulent quantities are influenced by kinetic energy gradients. In shear-less mixing, anisotropy is observed both at large and small scales, with significant intermittency in the velocity components along the mixing layer. This intermittency, marked by large maxima of skewness and kurtosis, is strongly dependent on energy and integral scale gradients. Notably, a variation in kinetic energy alone is enough to trigger intermittency.
Unlike homogeneous sheared turbulence or turbulence near fluctuating interfaces, shearless mixing produces unique turbulence structures. The turbulence energy gradient is externally imposed and does not intensify through the instability of mean shear. This leads to high intermittency fronts in the low-energy side of the flow, influenced by both large and small fluctuations. Our studies reveal that shearless mixing exhibits an intermediate form of anisotropy between isotropic turbulence and homogeneous sheared turbulence, where longitudinal derivative moments are the most significant. This provides new insights into the behavior of turbulent flows, offering valuable contributions to the understanding of turbulence at both laboratory and numerical scales.
We have analyzed data from numerical simulations where the Navier-Stokes equations were solved in a parallelepiped domain using a fully dealiased Fourier-Galerkin pseudospectral spatial discretization (employing the 3/2 rule) and fourth-order explicit Runge-Kutta time integration. The initial conditions were generated by linearly matching two homogeneous and isotropic fields over a narrow region, with the size of this region being comparable to the flow’s integral scale.

Shear-less turbulent mixing. Visualization of the kinetic energy in the central part of the mixing in a plane (x, y1), (y2 = const). The higher energy region has a Taylor microscale Reynolds number equal to 150, the energy ratio is 6.7. For more details on the flow, see the paper in Physical Review Letters (2011)
The time evolution of the mixing layer can be seen in this Movie, which shows the kinetic energy in the first 6 eddy turnover times.
Shearless turbulent mixing: A visualization of kinetic energy in a plane.
The high-energy region exhibits a Taylor microscale Reynolds number of 45.
Energy ratio: 6.7 | Uniform integral scale (watch the full simulation in the video!)
Shear-less turbulent mixing. Visualization of the kinetic energy in a plane. The higher energy region has a Taylor microscale Reynolds number equal to 45.
– Energy ratio 6.7, uniform integral scale (see Movie)
Passive scalar diffusion through a kinetic energy gradient. The higher energy turbulent region (Reλ=150) is on the left of the image, the lower energy region is on the right
The time evolution of the scalar diffusion is shown in the following movies:
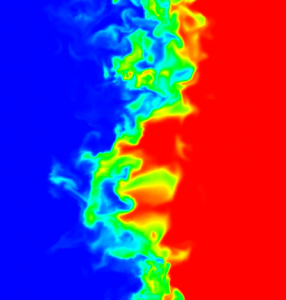
Passive scalar diffusion through a kinetic energy gradient in a two-dimensional flow. The higher energy turbulent region (Reλ=150) is on the left of the image, the lower energy region is on the right.
The time evolution of the scalar diffusion is shown by the following movies:
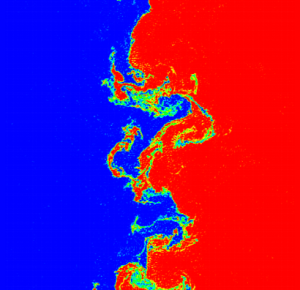
- 10242 simulation with hyperviscosity. See Movie
- 2048×4096 NS simulation, Rel=3500.
For more details on the flow, see JoT 2014 and the proceedings of ETC-13.
Kinetic energy profiles, comparison between the two-dimensional and the three-dimensional flow.
The black vertical lines indicate the borders of the mixing layers, and the vertical green line indicates the position of maximum intermittency
Vorticity field, two-dimensional simulation
Vorticity field in the presence of a stable stratification (Fr=10), preliminary result from a two-dimensional simulation
Vorticity field in the presence of a stable stratification (Fr=0.1), preliminary result from a two-dimensional simulation



